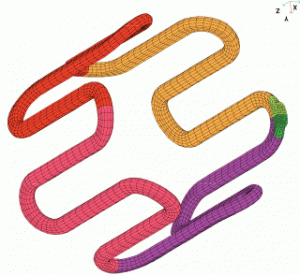
Finite Element Analysis (FEA) is a very general computer analysis technology that can be applied in a wide variety of industries and applications. The first step in the process involves “meshing” the geometry of the components – breaking them into small elements that represent the component geometry. Meshing may start with CAD geometry of the component or a dimensioned drawing describing the geometry. Even CT or laser scans of an actual may provide the starting geometry. Next material properties, loads, and boundary conditions are defined.
Linear analysis is often used for preliminary design assessments. PEC specializes in nonlinear analysis which permits more general and realistic simulations. Nonlinear analysis may include features such as nonlinear material properties, large displacement, large strain, contact, buckling, or material failure. More on nonlinear finite element analysis …



Finite Element Analysis of Composite CNG Fuel Tank Rear Impact
NGV_Rear_Impact
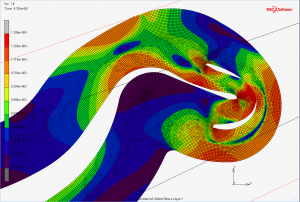
Fringe Plot of Plastic Strain during Impact
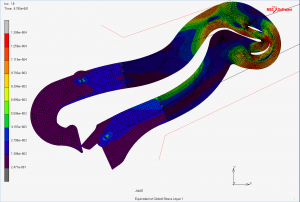
Fringe Plot of Total Displacement during Impact
CFD Introduction – Computational Fluid Dynamics
CFD_SCU_Fabris_01-intro1_2010